Standard Differentiable Structures
It’s high time we introduced the “standard” smooth structures on real vector spaces, which are (of course) our models for all other smooth manifolds.
The easiest one to discuss is 
. The standard smooth structure is given by starting with an extremely simple atlas: the single coordinate patch 
contains all of , and the coordinate map 
is just the identity! But we don’t just have this patch, of course. We also have all coordinate patches which are compatible with it.
Since the inverse 
is again the identity, it’s easy to pick these out. A coordinate patch is a subset 
and a real-valued function 
on 
. The two transition functions between and are 
and 
. Both of these functions must be differentiable for the patch to fit into the standard differentiable structure. They must both be smooth to fit into the standard smooth structure.
Now, consider a finite-dimensional real vector space . Again, the standard smooth structure starts with using all of as a coordinate patch. For the coordinate map, we can choose any linear isomorphism 
. Of course, we know that finding such a 
is equivalent to picking a basis — given a basis of we can just send it to the standard basis of 
, and given a linear isomorphism we can use the preimages of the standard basis to get a basis of .
So there’s a choice to be made: which linear isomorphism to start with. Does it matter? no! If 
and 
are two such linear isomorphisms, then 
is a linear automorphism on . And clearly this transition function is smooth. Thus all the possible choices are compatible with each other and generate the same smooth — and thus the same differentiable — structure.
We say “standard” structures here. This is because — and I know this might sound sort of hard to believe — there actually do exist “nonstandard” or “wild” differentiable structures as well. The proofs establishing these examples are tremendously complicated and I’m not about to go into them now. But the fact remains: there do exist manifolds which are homeomorphic to 
— they are equivalent to as topological spaces — and yet they are not equivalent as differentiable manifolds. Any homeomorphism from one topological space to the other will not be smooth.
March 2, 2011 -
Posted by John Armstrong |
Differential Topology, Topology
The Unapologetic Mathematician 
“Of course, we know that finding such a T is equivalent to picking a basis — given a basis of V we can just send it to the standard basis of \mathbb{R}^n, and given a linear isomorphism we can use the preimages of the standard basis to get a basis of V.”
This is where there seems to be a chicken/egg problem. It seems that the existence of the isomorphism T depends on already having a coordinate system in V, so what is the purpose of getting yet another coordinate system in V by using the preimage?
This converse pair is just a quick rundown of the equivalence that we’ve already pointed out before. It’s not that we’re trying to get new coordinate systems on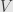
; we’re showing that the coordinate systems we’ve been using in linear algebra work as coordinate systems in differential topology and geometry. Further, we’re showing that they’re all compatible with each other as smooth coordinate maps.
[…] a smooth manifold is nothing but a smooth map , where is some interval in the real line with its standard differentiable structure. The interval can, in principle, be half-infinite or infinite, but commonly we just consider […]
Pingback by Curves « The Unapologetic Mathematician | April 8, 2011 |
[…] bundle to a Euclidean space. That is, we let be a finite-dimensional real vector space with its standard differentiable structure and see what these constructions look […]
Pingback by The Tangent Bundle of a Euclidean Space « The Unapologetic Mathematician | April 11, 2011 |
[…] we can conclude that is an open submanifold of , which comes equipped with the standard differentiable structure on . Matrix multiplication is clearly smooth, since we can write each component of a product matrix […]
Pingback by General Linear Groups are Lie Groups « The Unapologetic Mathematician | June 9, 2011 |