A note on the Periodic Functions Problem
Over at The Everything Seminar, Jim Belk mentions an interesting little problem.
Show that there exist two periodic functions 
whose sum is the identity function:
for all 
He notes right off that, “Obviously the functions 
and 
can’t be continuous, since any continuous periodic function is bounded.” I’d like to explain why, in case you didn’t follow that.
If a function is periodic, that means it factors through a map to the circle, which we call 
. Why? Because “periodic” with period 
means we can take the interval 
and glue one end to the other to make a circle. As we walk along the real line we walk around the circle. When we come to the end of a period in the line, that’s like getting back to where we started on the circle. Really what we’re doing is specifying a function on the circle and then using that function over and over again to give us a function on the real line. And if is going to be continuous, the function 
had better be as well.
Now, I assert that the circle is compact. I could do a messy proof inside the circle itself (and I probably should in the long run) but for now we can just see the circle lying in the plane 
as the collection of points distance 
from the origin. Then this subspace of the plane is clearly bounded, and it’s not hard to show that it’s closed. The Heine-Borel theorem tells us that it’s compact!
And now since the circle is compact we know that its image under the continuous map 
must be compact as well! And since the image of is the same as the image of , it must also be a compact subspace of 
— a closed, bounded interval. Neat.
January 23, 2008 -
Posted by John Armstrong |
Analysis, Calculus
The Unapologetic Mathematician 
It looks like you are using heavy machinery where some hand tools are enough. A continuous function over any closed interval is bounded, in particular, any continuous periodic function is.
But how do you know that a continuous function on a closed interval is bounded?
Suppose it’s unbounded, then there is a sequence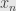
such that 
. We may assume that 
, otherwise take a convergent subsequence. By continuity we must have 
, a contradiction.
And you have a convergent subsequence by compactness. You’re actually reproving parts of the theorems I quoted but now in the language of sequential compactness (which is equivalent to compactness in metric spaces).
Yes, I’m using a different viewpoint than an advanced calculus textbook would, emphasizing the point-set topology underlying the analysis. Besides, I stumbled across something on another weblog that related back to my recent posts, so why not show the connection?
Here is another proof that is not using compactness. Let our function f be defined on [a,b], and let c be the lowest upper bound of such t that f is bounded on [a,t]. Assume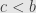
, then f is bounded (by continuity at c) on some open interval (c-e,c+e), and also bounded on [a,c-e], therefore it’s bounded on [a,c+e/2], and c is not an upper bound.
Which is exactly the same method I used to prove that an interval is compact.
I guess your blog rubs me the wrong way because of too many complicated explanations of simple things and too few simple explanations of complicated things. It gives people rather cartoonish biew of mathematics. And by the way, do you plan to explain the topological meaning of gluing one end of a segment to the other, while you are still at it?
That’s a quotient space, as I described them earlier.
I’m not intending this to be a textbook so much as an overview, showing a certain natural flow emphasizing common themes throughout. It’s not a palette, it’s a painting.
Though there is a fair amount of heavy machinery involved in the posts, I do think that a more “general” approach to analysis is important in developing an understanding of the subject. And, emphasizing a point-set topological foundation of analysis goes a long way in “seeing” what’s actually going on.
Though there is a fair amount of heavy machinery involved in the posts, I do think that a more “general” approach to analysis is important in developing an understanding of the subject. And, emphasizing a point-set topological foundation of analysis goes a long way in “seeing” what’s actually going on.
John, this is one of the few places I know where it is possible to get an idea of how these many complex pieces fit together without spending a lifetime learning all the details about the pieces. Your blog provides motivation. It is balanced. It greatly facilitates a more detailed study, should that be desired. Clearly communicating ideas (even simple ones, let alone complex ones!) is one of the most difficult tasks we face; out of hundreds of people who “understand” something, we are lucky indeed to find even one who is willing and able to clearly explain it. Creating and maintaining this blog is a difficult task and a significant commitment. Know that it is greatly appreciated! Thank you!
I’d echo Charlie C’s comment. The style of this blog is great for people who have yet to come across material being mentioned and is quite motivating in that regard.
I sometimes read posts here and don’t take the time to follow/understand them fully but just take the gist of it and try and then hopefully when I seriously study the aforementioned topic at least it will seem faintly familiar.
[…] need to get down a few facts about metric spaces before we can continue on our course. Firstly, as I alluded in an earlier comment, compact metric spaces are sequentially compact — every sequence has a convergent […]
Pingback by Some theorems about metric spaces « The Unapologetic Mathematician | January 31, 2008 |