The Zero Representation
Okay, this is going to sound pretty silly and trivial, but I’ve been grading today. There is one representation we always have for any group, called the zero representation 
.
Pretty obviously this is built on the unique zero-dimensional vector space . It shouldn’t be hard to convince yourself that 
is the trivial group, and so any group 
has a unique homomorphism to this group. Thus there is a unique representation of on the vector space .
We should immediately ask: is this representation a zero object? Suppose we have a representation 
. Then there is a unique arrow 
sending every vector 
to 
. Similarly, there is a unique arrow 
sending the only vector to the zero vector 
. It’s straightforward to show that these linear maps are intertwinors, and thus that the zero representation is indeed a zero object for the category of representations of .
This is all well and good for groups, but what about representing an algebra 
? This can only make sense if we allow rings without unit, which I only really mentioned back when I first defined a ring. This is because there’s only one endomorphism of the zero-dimensional vector space at all! The endomorphism algebra will consist of just the element 
, and a representation of has to be an algebra homomorphism to this non-unital algebra. Given this allowance, we do have the zero representation, and it’s a zero object just as for groups. It’s sort of convenient, so we’ll tacitly allow this one non-unital algebra to float around just so we can have our zero representation, even if we allow no other algebras without units.
December 8, 2008 -
Posted by John Armstrong |
Algebra, Representation Theory
The Unapologetic Mathematician 
There is nothing in the definition of rings with unit that demands that the unit be distinct from 0. (Or if there is, it’s the wrong definition!) Indeed, the theory of unital rings is equational, and the condition that 1 not equal 0 is a non-equational condition; demanding it would be somewhat wrong-headed from the categorical and logical points of view.
Moral: the endomorphism algebra on the zero vector space may be considered unital.
I suppose there’s a point to that. I just see every time someone says “unital” they’re very emphatic that it’s not zero.
The only case I can remember such an explicit emphasis is for the case of fields, where the convention would be I think more defensible (since, e.g., already the theory of fields is non-equational in the sense we mean, i.e., Lawvere theories). If I saw it in the more general case of rings, I’d be tempted to write the author!
0 is indeed a unital ring, but it isn’t a zero object in the category of unital rings.
Consider a morphism f with domain 0 and codomain an algebra A. f(0) = 0 and (if f is unital) f(1) = 1, and 0 = 1 in the zero ring; so 0 = 1 in A, and A must be the zero ring, otherwise no such morphism exists.
Whether that matters for your purposes, I don’t know.
It doesn’t. I’m making the vector space a zero object, not its endomorphism ring.
Todd, Chad kind of nailed it. The zero ring may be a ring with unit, but when you’re talking about rings with unit, you tend to require that the homomorphisms preserve the unit, which isn’t viable here.
Charles, no. The “here” in question is an algebra morphism going the other way: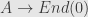
. The unit is preserved.
It’s perfectly fine that there be no algebra homomorphism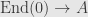
unless 
is also the one-element ring. Looked at from the point of view of the opposite category, affine spectra, that’s like saying there’s no morphism 
to the empty spectrum, unless 
is itself empty. Which is a general feature of what are called distributive categories.
Hm, obviously the bit about the opposite category pertains to commutative unital rings. Not that that affects any of what we’re talking about here.
Incidentally, I like the convention of calling rings not assumed as carrying units “rngs”: rings without the i-dentity. I think the term is due to Jacobson.
Yes, Todd’s right. I’m not trying to make the homomorphism go back the other way. Remember, the homomorphism of rings is the representation, which doesn’t have to go back the other way.
And Chad’s right that the zero ring is not a zero object in the category of unital rings. But I’m not trying to find a zero object in that category.
As for rngs.. what happens if you throw away negatives as well? Say, the strictly positive natural numbers? Are they a “rg”?
Yes, there seems to be some scholarly controversy about that: whether those ancient Indian treatises should be rendered as the Rig Vedas or the Rg Vedas.
(Seriously, there are such things as rigs = rings without n-egatives = monoids in the monoidal category of commutative monoids, as John already knows I’m sure. I’ve never seen rgs mentioned though. How would you even pronounce that: “erg”?)
Well, yes, I know that there are rigs. That’s the point: take away identity and you have rng. Take away negatives and you have rig. Take away *both*?
Yes, I knew what you meant. But I’ve never seen them mentioned by anyone until now.