Parallelepipeds and Volumes I

And we’re back with more of what Mr. Martinez of Harvard’s Medical School assures me is onanism of the highest caliber. I’m sure he, too, blames me for not curing cancer.

Coming up in our study of calculus in higher dimensions we’ll need to understand parallelepipeds, and in particular their volumes. First of all, what is a parallelepiped? Or, more specifically, what is a -dimensional parallelepiped in -dimensional space? It’s a collection of points in space that we can describe as follows. Take a point and vectors in . The parallelepiped is the collection of points reachable by moving from by some fraction of each of the vectors . That is, we pick values , each in the interval , and use them to specify the point . The collection of all such points is the parallelepiped with corner and sides .

One possible objection is that these sides may not be linearly independent. If the sides are linearly independent, then they span a -dimensional subspace of the ambient space, justifying our calling it -dimensional. But if they’re not, then the subspace they span has a lower dimension. We’ll deal with this by calling such a parallelepiped “degenerate”, and the nice ones with linearly independent sides “nondegenerate”. Trust me, things will be more elegant in the long run if we just deal with them both on the same footing.

Now we want to consider the volume of a parallelepiped. The first observation is that the volume doesn’t depend on the corner point . Indeed, we should be able to slide the corner around to any point in space as long as we bring the same displacement vectors along with us. So the volume should be a function only of the sides.

The second observation is that as a function of the sides, the volume function should commute with scalar multiplication in each variable separately. That is, if we multiply by a non-negative factor of , then we multiply the whole volume of the parallelepiped by as well. But what about negative scaling factors? What if we reflect the side (and thus the whole parallelepiped) to point the other way? One answer might be that we get the same volume, but it’s going to be easier (and again more elegant) if we say that the new parallelepiped has the negative of the original one’s volume.

Negative volume? What could that mean? Well, we’re going to move away from the usual notion of volume just a little. Instead, we’re going to think of “signed” volume, which includes the possibility of being positive or negative. By itself, this sign will be less than clear at first, but we’ll get a better understanding as we go. As a first step we’ll say that two parallelepipeds related by a reflection have opposite signs. This won’t only cover the above behavior under scaling sides, but also what happens when we exchange the order of two sides. For example, the parallelogram with sides and and the parallelogram with sides and have the same areas with opposite signs. Similarly, swapping the order of two sides in a given parallelepiped will flip its sign.

The third observation is that the volume function should be additive in each variable. One way to see this is that the -dimensional volume of the parallelepiped with sides through should be the product of the -dimensional volume of the parallelepiped with sides through and the length of the component of perpendicular to all the other sides, and this length is a linear function of . Since there’s nothing special here about the last side, we could repeat the argument with the other sides.

The other way to see this fact is to consider the following diagram, helpfully supplied by Kate from over at f(t):

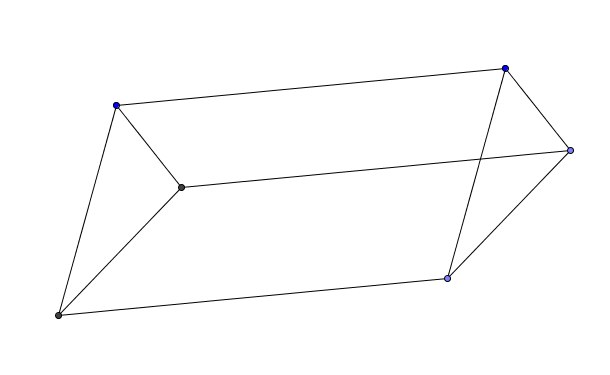
The side of one parallelogram is the (vector) sum of the sides of the other two, and we can see that the area of the one parallelogram is the sum of the areas of the other two. This justifies the assertion that for parallelograms in the plane, the area is additive as a function of one side (and, similarly, of the other). Similar diagrams should be apparent to justify the assertion for higher-dimensional parallelepipeds in higher-dimensional spaces.

Putting all these together, we find that the -dimensional volume of a parallelepiped with sides is an alternating multilinear functional, with the sides as variables, and so it lives somewhere in the exterior algebra . We’ll have to work out which particular functional gives us a good notion of volume as we continue.