Outer Measures
We’re going to want a modification of the notion of a measure. But before we introduce it, we have (of course) a few definitions.
First of all, a collection 
of sets is called “hereditary” if it includes all the subsets of each of its sets. That is, if 
and 
, then 
as well. It’s not very useful to combine this with the definition of an algebra, because an algebra must contain 
itself; the only hereditary algebra is 
itself. Instead, we define a “ring” of sets (or a 
-ring) to be closed under union (countable unions for -rings) and difference operations, but without the requirement that it contain ; complements and intersections are also not guaranteed, since we built these from differences using itself. Pretty much everything we’ve done so far with algebras can be done with rings, and hereditary -rings will be interesting objects of study.
Just like we found for algebras and monotone classes, the intersection of two hereditary collection is again hereditary. We can thus construct the “smallest” hereditary -ring containing a given collection 
, and we’ll call it 
. In fact, it’s not hard to see that this is the collection of all sets which can be covered by a countable union of sets in ; any -ring containing must contain all such countable unions, and a hereditary collection must then contain all the subsets.
Now, an extended real-valued set function 
on a collection is called “subadditive” whenever 
, 
, and their union 
are in , we have the inequality
It’s called “finitely subadditive” if for every finite collection 
whose union is also contained in we have the inequality
and “countably subadditive” if for every sequence 
of sets in whose union is also in , we have
Note that these differ from additivity conditions in two ways: we only ask for an inequality to hold, and we don’t require the unions to be disjoint.
Finally, we can define an “outer measure” to be an extended real-valued, non-negative, monotone, and countably subadditive set function , defined on a hereditary -ring 
, and such that 
. Just as for a measure, we say that is “finite” or “-finite” if every set has finite or -finite outer measure.
March 25, 2010 -
Posted by John Armstrong |
Analysis, Measure Theory
The Unapologetic Mathematician 
Hi,
I used to see Lebesgue measure and Lebesgue outer measure, so somehow I thought that whenever we define outer measure, we are looking for a monotone countably subadditive function on the power set of X. Why would we want to put this setting on hereditary sigma rings (instead on the power set) and is there any motivation for its definition? Thanks!
[…] to an Outer Measure Let be a measure in a ring (not necessarily an algebra) , and let be the hereditary -ring generated by . For every , […]
Pingback by Extending a Measure to an Outer Measure « The Unapologetic Mathematician | March 26, 2010 |
[…] Measurable by an Outer Measure I An outer measure on a hereditary -ring is nice and all, but it’s not what we really want, which is a […]
Pingback by Sets Measurable by an Outer Measure I « The Unapologetic Mathematician | March 29, 2010 |
Soarer, like I said above there’s only one hereditary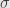
–algebra: 
. But much of measure theory can be done with just unions and differences, so a ring is all the structure we really need.
[…] Measurable by an Outer Measure II Yesterday, we showed that — given an outer measure on a hereditary -ring — the collection of -measurable sets forms a ring. In fact, it forms […]
Pingback by Sets Measurable by an Outer Measure II « The Unapologetic Mathematician | March 30, 2010 |
[…] and Induced Measures If we start with a measure on a ring , we can extend it to an outer measure on the hereditary -ring . And then we can restrict this outer measure to get an actual measure on […]
Pingback by Measures and Induced Measures « The Unapologetic Mathematician | March 31, 2010 |
[…] analogy with the outer measure induced on the hereditary -ring by the measure on the -ring , we now define the “inner […]
Pingback by Inner Measures « The Unapologetic Mathematician | April 8, 2010 |
[…] covers and measurable kernels. These will allow us to move statements we want to show about outer and inner measures to the realm of proper measures, where we can use nice things like […]
Pingback by Using Measurable Covers and Kernels I « The Unapologetic Mathematician | April 12, 2010 |
[…] of a set to be the infimum of the volumes of finite open covers. And, indeed, this is exactly the outer measure corresponding to Lebesgue measure […]
Pingback by Borel Sets and Lebesgue Measure « The Unapologetic Mathematician | April 20, 2010 |
[…] . Given a subset , we write for the image of under the transformation — . I say that the outer and inner Lebesgue measures are both nicely behaved under the transformation […]
Pingback by Lebesgue Measure and Affine Transformations « The Unapologetic Mathematician | April 22, 2010 |
[…] let’s recall where they come from. There’s an outer measure associated with Lebesgue measure , and then there is the collection of sets measurable by . These […]
Pingback by Lebesgue Measurable Sets « The Unapologetic Mathematician | April 23, 2010 |
[…] the measurable space. This is not to insinuate that is the collection of sets measurable by some outer measure , nor even that we can define a nontrivial measure on in the first place. Normally we just call […]
Pingback by Measurable Spaces, Measure Spaces, and Measurable Functions « The Unapologetic Mathematician | April 26, 2010 |