Tangent Vectors at a Point
Tangent vectors are a very important concept in differential geometry, and they’re one of the biggest stumbling blocks in comprehension. There are two major approaches: one more geometric, and one more algebraic. I find the algebraic approach a bit more satisfying, since it gets straight into the important properties of tangent vectors and how they are used, and it helps set the stage for tangent vectors in other contexts like algebraic geometry. Unfortunately, it’s not at all clear at first what this definition means geometrically, and why these things deserve being called “tangent vectors”. So I have to ask a little patience.
Now, we take a manifold with structure sheaf
. We pick some point
and get the stalk
of germs of functions at
. This is a real algebra, and we define a “tangent vector at
” to be a “derivation at
” of this algebra. That is,
is a function
satisfying
The first of these conditions says that is a linear functional on
. It’s the second that’s special: it tells us that
obeys something like the product rule.
Indeed, let’s take a point and consider the operation
defined by
for any function
that is differentiable at
. This is linear, since both the derivative and evaluation operations are linear. The product rule tells us that
So satisfies the definition of a “tangent vector at
“. Indeed, as it turns out
corresponds to what we might normally consider the vector based at
pointing one unit in the positive direction.
It should immediately be clear that the tangent vectors at form a vector space. Indeed, the sum of two tangent vectors at
is firstly the sum of two linear functionals, which is again a linear functional. To see that it also satisfies the “derivation” condition, let
and
be tangent vectors at
and check
Checking that scalar multiples of tangent vectors at are again tangent vectors at
is similar. We write
to denote this vector space of tangent vectors at
to the manifold
.
I want to call attention to one point of notation here, and I won’t really bother with it again. We seem to be using each of and
to refer to two different things: a germ in
— which is an equivalence class of sorts — and some actual function in
for some neighborhood
of
which represents the germ. To an extent we are, and the usual excuse is that since we only ever evaluate the function at
itself, it doesn’t really matter which representative of the germ we pick.
However, a more nuanced view will see that we’ve actually overloaded the notation . Normally this would mean evaluating a function at a point, yes, but here we interpret it in terms of the local ring structure of
. Given a germ
there is a projection
, which we write as
.
If all this seems complicated, don’t really worry about it. You can forget the whole last paragraph and get by on “sometimes we use a germ as if it’s an actual function defined in a neighborhood of , and it will never matter which specific representative function we use because we only ever ask what happens at
itself.”

[…] « Previous | […]
Pingback by Tangent Vectors and Coordinates « The Unapologetic Mathematician | March 30, 2011 |
[…] a point in an -dimensional manifold , we have the vector space of tangent vectors at . Given a coordinate patch around , we’ve constructed coordinate vectors at , and shown […]
Pingback by Coordinate Vectors Span Tangent Spaces « The Unapologetic Mathematician | March 31, 2011 |
Should the second equation have on its lhs?
on its lhs?
Or perhaps a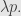 on its rhs
on its rhs
No, I go into this down near the bottom. Since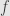 and
and  are germs at
are germs at 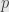 , they each have a “value at
, they each have a “value at 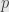 “, which we write
“, which we write 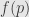 and
and  .
.
Everything in sight is taking place “at” the single point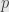 here. A tangent vector
here. A tangent vector  at
at 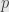 takes a germ
takes a germ 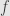 at
at 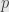 and gives a real number.
and gives a real number.
[…] of a point in an -dimensional manifold , we get coordinate vectors which form a basis for the tangent space . But this is true of any coordinate patch! If we have another patch , we can get another basis . […]
Pingback by Coordinate Transforms on Tangent Vectors « The Unapologetic Mathematician | April 1, 2011 |
[…] far we’ve talked about tangent spaces one at a time. For each we get a tangent space at . But things get really interesting when we […]
Pingback by The Tangent Bundle « The Unapologetic Mathematician | April 4, 2011 |
[…] any point of an open interval, the tangent space is one-dimensional. And, in fact, it comes equipped with a canonical vector to use as a basis: , […]
Pingback by Curves « The Unapologetic Mathematician | April 8, 2011 |
There is an obvious typo in the first equation (you have a where you meant
where you meant 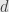 ).
).
Thanks, fixed.
[…] another construct in differential topology and geometry that isn’t quite so obvious as a tangent vector, but which is every bit as useful: a cotangent vector. A cotangent vector at a point is just an […]
Pingback by Cotangent Vectors, Differentials, and the Cotangent Bundle « The Unapologetic Mathematician | April 13, 2011 |
[…] manifolds, with the -dimensional product manifold. Given points and we want to investigate the tangent space of this product at the point […]
Pingback by The Tangent Space of a Product « The Unapologetic Mathematician | April 27, 2011 |
[…] with boundary , then at all the interior points it looks just like a regular manifold, and so the tangent space is just the same as ever. But what happens when we consider a point […]
Pingback by The Tangent Space at the Boundary « The Unapologetic Mathematician | September 15, 2011 |