The Classification of (Possible) Root Systems

At long last, we can state the classification of irreducible root systems up to isomorphism. We’ve shown that for each such root system we can construct a connected Dynkin diagram, which determines a Cartan matrix, which determines the root system itself, up to isomorphism. So what he have to find now is a list of Dynkin diagrams which can possibly arise from a root system. And so today we state the

CLASSIFICATION THEOREM

If $\Phi$ is an irreducible root system, then its Dynkin diagram is in one of four infinite families, or one of five “exceptional” diagrams. The four infinite families are

The $A$ series. These consist of a single long string of roots with single edges connecting them. The diagrams are indexed by the number of roots, starting at $n=1$ :

$A_1$ :
$A_2$ :
$A_3$ :
$A_4$ :
$A_5$ :
$A_6$ :
$A_n$ :

The $B$ series. These look like the $A$ series, except the last two roots are connected by a double edge, with the last root shorter. The diagrams are indexed by the number of roots, starting at $n=2$ :

$B_2$ :
$B_3$ :
$B_4$ :
$B_5$ :
$B_6$ :
$B_n$ :

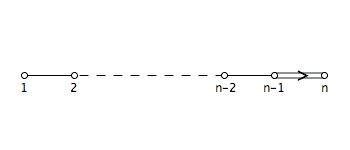
The $C$ series. These look like the $B$ series, except the last root is longer. The diagrams are indexed by the number of roots, starting at $n=3$ :

$C_3$ :
$C_4$ :
$C_5$ :
$C_6$ :
$C_n$ :

The $D$ series. These look like the $A$ series, except the end is split into two roots. The diagrams are indexed by the number of roots, starting at $n=4$ :

$D_4$ :
$D_5$ :
$D_6$ :
$D_7$ :
$D_n$ :

The exceptional diagrams are

The $E$ series. These have a string of roots connected by single edges. On the third root from the end of the string, a single edge branches off to another root on the side. The diagrams are indexed by the number of roots, for $n=6$ , $n=7$ , and $n=8$ :

$E_6$ :
$E_7$ :
$E_8$ :

The $F_4$ diagram. This consists of four roots in a row. The first two and last two are connected by a single edge, while the middle two are connected by a double edge:

$F_4$ :

The $G_2$ diagram. This consists of two roots, connected by a triple edge:

$G_2$ :

Our proof will take a number of steps, winnowing down the possible diagrams to this collection. We will spread it over the next week. But over the weekend, you can amuse yourself by working out the Cartan matrices for each of these Dynkin diagrams.

February 19, 2010 - Posted by John Armstrong | Geometry, Root Systems

12 Comments »

OK, I’m going to have to reread this sequence on root systems again, and more carefully. When I saw your classification of root systems into 4 infinite families and 5 exceptions, my mind went “hey, those look suspiciously like the classification of Lie groups I barely understand…”. Wikipedia confirms that suspicion.

I wondered where you were going with the root systems stuff.

Comment by Blaise Pascal | February 19, 2010 | Reply
Root systems come up in many places, Blaise, and can be motivated and described just in terms of high school notions of spatial transformations. But I might talk about Lie groups eventually 😀

Comment by John Armstrong | February 19, 2010 | Reply
At long last, as we’ve been thinkin’, we come to Dynkin.

Comment by Jonathan Vos Post | February 20, 2010 | Reply
[…] the Classification Theorem I This week, we will prove the classification theorem for root systems. The proof consist of a long series of steps, and we’ll split it up over a […]

Pingback by Proving the Classification Theorem I « The Unapologetic Mathematician | February 22, 2010 | Reply
[…] the Classification Theorem II We continue with the proof of the classification theorem that we started […]

Pingback by Proving the Classification Theorem II « The Unapologetic Mathematician | February 23, 2010 | Reply
[…] continue with the proof of the classification theorem. The first two parts are here and […]

Pingback by Proving the Classification Theorem III « The Unapologetic Mathematician | February 25, 2010 | Reply
[…] the Classification Theorem IV We continue proving the classification theorem. The first three parts are here, here, and […]

Pingback by Proving the Classification Theorem IV « The Unapologetic Mathematician | February 25, 2010 | Reply
[…] the Classification Theorem V Today we conclude the proof of the classification theorem. The first four parts of the proof are here, here, here, and […]

Pingback by Proving the Classification Theorem V « The Unapologetic Mathematician | February 26, 2010 | Reply
[…] of Root Systems (setup) Now that we’ve proven the classification theorem, we know all about root systems, right? No! All we know is which Dynkin diagrams could […]

Pingback by Construction of Root Systems (setup) « The Unapologetic Mathematician | March 1, 2010 | Reply
[…] at the Dynkin diagram! Let’s see what this looks like for the connected diagrams in the classification theorem, since disconnected diagrams just add transformations that shuffle isomorphic […]

Pingback by The Automorphism Group of a Root System « The Unapologetic Mathematician | March 11, 2010 | Reply
[…] we could start the real work of classification: a list of the Dynkin diagrams that might arise from root systems. And then we could actually construct […]

Pingback by Root Systems Recap « The Unapologetic Mathematician | March 12, 2010 | Reply
Awesome stuff! I have benefited immensely from your posts, thank you for all the work you have put in.

Comment by Sangeeta Bhatia | July 27, 2012 | Reply

About this weblog

This is mainly an expository blath, with occasional high-level excursions, humorous observations, rants, and musings. The main-line exposition should be accessible to the “Generally Interested Lay Audience”, as long as you trace the links back towards the basics. Check the sidebar for specific topics (under “Categories”).

I’m in the process of tweaking some aspects of the site to make it easier to refer back to older topics, so try to make the best of it for now.

RSS Feeds

RSS - Posts
RSS - Comments
Feedback

Got something to say? Anonymous questions, comments, and suggestions at Formspring.me!
Subjects

Subjects
Archives

February 2010

M T W T F S S

1 2 3 4 5 6 7

8 9 10 11 12 13 14

15 16 17 18 19 20 21

22 23 24 25 26 27 28

« Jan Mar »
Search for:

The Unapologetic Mathematician

Mathematics for the interested outsider