Adjoints with parameters
Now that we know how to transform adjoints, we can talk about whole families of adjoints parametrized by some other category. That is, for each object of the parametrizing category
we’ll have an adjunction
, and for each morphism of
we’ll have a transformation of the adjunctions.
Let’s actually approach this from a slightly different angle. Say we have a functor , and that for each
the functor
has a right adjoint
. Then I claim that there is a unique way to make
into a functor from
to
so that the bijection
is natural in all three variables. Note that
must be contravariant in
here to make the composite functors have the same variance in
.
If we hold fixed, the bijection is already natural in
and
. Let’s hold
and
fixed and see how to make it natural in
. The components
are already given in the setup, so we can’t change them. What we need are functions
and
for each arrow
.
For naturality to hold, we need . But from what we saw last time this just means that the pair of natural transformations
forms a conjugate pair from
to
. And this lets us define
uniquely in terms of
, the counit
of
, and the unit
of
by using the first of the four listed equalities.
From here, it’s straightforward to show that this definition of how acts on morphisms of
makes it functorial in both variables, proving the claim. We can also flip back to the original viewpoint to define an adjunction between categories
and
parametrized by the category
as a functor from
to the category
of adjunctions between those two categories.

With every new post you make I’m finding out that I know almost nothing about adjoints!
Do you plan on talking about fibered categories at some point?
Maybe eventually I will. I’ve got a short-term goal for the category theory, though, before I can finally move on to topology.
And analysis.
And linear algebra.
And representation theory…
Suppose C is a monoidal category and F is the monoidal operation. Suppose further that the functor F(_,P) has a right adjoint, g(P) (contravariant) for each object P in C.
Now it seems that you are suggesting that there is a functor
G: F(C,C)–>C such that g(P) = G(P,_)
Assuming that I am understanding you correctly,
1) Can G be thought of as a right adjoint to F?
2) Is G(P,Q) necessarily an (the) exponential object Q^P? If it is, then C is, by definition, a closed category, right?
3) Can one start with a category C, a bifunctor G and define another functor F analogously? Presumably, G would have to satisfy properties similar to the monoidal operation but not quite dual properties.
dan
Dan, I think your notation is a bit confused, but I can answer question 2 definitely. Yes, this is the definition of the exponential object, and in fact that’s why I gave this definition. If has a right adjoint
has a right adjoint 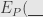 for all
for all  , then these right adjoints fit together to make a functor
, then these right adjoints fit together to make a functor 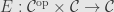 so that
so that  .
.
In this case, the functor isn’t the “right adjoint”, but the two functors together define a parametrized family of adjunctions.
And yes, you can start with something like the exponential and extract a monoidal structure given the correct left adjoints. In fact, I did just this just the other day when I talked about ordered linear spaces.
Of course, neither of these families of adjoints has to exist. But when they do they give us a lot of useful structure to work with.