The 2-category of Enriched Categories
So we know that 
— the collection of all categories — forms a 2-category with functors as 1-morphisms and with natural transformations as 2-morphisms. It shouldn’t surprise us, then, that the collection 
of all categories enriched over a monoidal category 
also comprises a 2-category.
We need two concepts to make this go through: an “enriched” notion of a functor, and an “enriched” notion of a natural transformation. As we might expect, both of them will just be written out like the familiar notions of functor and natural transformation, but substituting the new monoidal structure for the cartesian monoidal structure of 
.
First of all, a functor from category 
to 
is a function 
, along with functions 
for each hom-set. So to define a -functor we keep the function on objects and replace the functions between hom-sets with morphisms between hom-objects. Of course, these must preserve compositions and identities, as encoded in the following diagrams:
which by now should look very familiar.
A natural transformation 
between two functors from to picks out a morphism 
in for each object 
in , subject to a “naturality” condition. To find an analogue of picking out a morphism from a hom-set we use the same trick we did for the identity: we pick a morphism from 
to a hom-object. That is, a -natural transformation consists of an 
-indexed family of arrows 
, which make the following diagram commute:
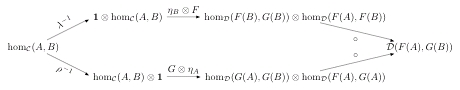
You should try to write this diagram out in the case of to verify that it becomes the familiar naturality square in that context.
Now the exact same constructions we used to compose natural transformations “vertically” and “horizontally” apply to -natural transformations, and the same arguments we used in the case of apply to give a 2-category of categories, functors, and natural transformations, all enriched over the monoidal category .
August 17, 2007 -
Posted by John Armstrong |
Category theory
The Unapologetic Mathematician 
So we have 2-categories, and we also have mondoidal 2-categories (Karpanov and Voevodsky paper, or else follow along in Leinster’s book and view monoidal 2-categories as three-categories with a single object). Perhaps you have a pithy way of describing the diagrams needed for monoidal 2-functor?
I’m not sure what you mean by “pithy”.
It partly depends on what you mean by monoidal 2-category; the way you write suggests you mean the weakest notion — that of tricategory with one object — but a reasonable semi-strictified alternative would be a Gray monoid (monoid enriched in the category of 2-categories equipped with the “pseudo” version of Gray’s tensor product).
I suggest just looking it up. If memory serves, you can find the weak version in Day and Street, Monoidal bicategories and Hopf algebroids, Adv. Math. 129 (1997), 99-157.
But if you want “pith”: the combinatorics of such diagrams appear from long ago in the study of
maps between 
spaces, by people such as Stasheff, Boardman and Vogt, and others, and can be described in terms of combinatorics of “cherry trees” (Boardman and Vogt). A key word here is “multiplihedra”, and you may wish to check out some of the work of Stefan Forcey and his collaborators, as for example here. A modern understanding of these structures would be in terms of bimodules between operads, but the margin here is not really wide enough to go into details.