The Interior Product
We have yet another operation on the algebra 
of differential forms: the “interior product”. Given a vector field 
and a 
-form 
, the interior product 
is the 
-form defined by
=\omega(X,X_1,\dots,X_{k-1})](https://s0.wp.com/latex.php?latex=%5Cdisplaystyle%5Cleft%5B%5Ciota_X%5Comega%5Cright%5D%28X_1%2C%5Cdots%2CX_%7Bk-1%7D%29%3D%5Comega%28X%2CX_1%2C%5Cdots%2CX_%7Bk-1%7D%29&bg=e6e6e6&fg=333333&s=0&c=20201002)
That is, we just take the vector field 
and stick it into the first “slot” of a -form. We extend this to functions by just defining 
.
Two interior products anticommute: 
, which follows easily from the antisymmetry of differential forms. Each one is also clearly linear, and in fact is also a graded derivation of with degree -1:
where 
is the degree of 
. This can be shown by reducing to the case where and 
are wedge products of 
-forms, but rather than go through all that tedious calculation we can think about it like this: sticking into a slot of 
means either sticking it into a slot of or into one of . In the first case we just get 
, while in the second we have to “move the slot” through all of , which incurs a sign of 
, as asserted.
July 26, 2011 -
Posted by John Armstrong |
Differential Topology, Topology
The Unapologetic Mathematician 
Why is this post in the “point-set topology” category?
An errant click on a very tightly-spaced list.
[…] turns out that there is a fantastic relationship between the interior product, the exterior derivative, and the Lie […]
Pingback by Cartan’s Formula « The Unapologetic Mathematician | July 26, 2011 |
[…] along the projection to get a “time” vector field on the cylinder. Then we use the interior product to assert that and […]
Pingback by The Poincaré Lemma (proof) « The Unapologetic Mathematician | December 3, 2011 |
[…] and applying it to the vector itself: . We also take the canonical volume form , and we use the interior product to define the -form […]
Pingback by A Family of Nontrivial Homology Classes (part 1) « The Unapologetic Mathematician | December 20, 2011 |
hi thank you for this post.
when we insert X into a slot of say a, then don’t we need to move X so that it will be on the first slot of a? And this will require multiplying by (-1) times the number of swaps.
That’s true, circa1687. Think of it like this: because of antisymmetry, the interior product doesn’t really care which slot you insert
into, since it just picks up a sign as you move it to the front. Inserting into the second slot of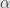 
is the same as inserting it into the second slot of 
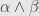, but inserting into the second slot of 
is like inserting it into the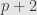 
th slot of 
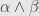, so we always pick up an extra 
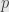signs.