The Riemann-Stieltjes Integral II

What with cars not working right, I’m not along with the undergraduate math club at the MAA meeting in Lake Charles. That honor goes to Steve Sinnott alone. And so I’m still back in New Orleans, and I may as well write another post today.

Let’s follow on yesterday’s discussion of the Riemann-Stieltjes integral by looking at a restricted sort of integrator. We’ll assume here that $\alpha(t)$ is continuously differentiable — that is, that $\alpha'(t)$ exists and is continuous in the region we care about. We’ll also have a function $f$ on hand to integrate.

Now let’s take a tagged partition $x=((x_0,...,x_n),(t_1,...,t_n))$ and set up our Riemann-Stieltjes sum for $f$

$\displaystyle f_{\alpha,x}=\sum\limits_{i=1}^nf(t_i)(\alpha(x_i)-\alpha(x_{i-1}))$

We can also use the partition to set up a Riemann sum for $f\alpha'$

$\displaystyle(f\alpha')_x=\sum\limits_{i=1}^nf(t_i)\alpha'(t_i)(x_i-x_{i-1})$

Now the Differential Mean Value Theorem tells us that in each subinterval $\left[x_{i-1},x_i\right]$ there is some $s_i$ so that $(\alpha(x_i)-\alpha(x_{i-1})=\alpha'(s_i)(x_i-x_{i-1})$ . We stick this into the Riemann-Stieltjes sum and subtract from the Riemann sum

$\displaystyle(f\alpha')_x-f_{\alpha,x}=\sum\limits_{i=1}^nf(t_i)(\alpha'(t_i)-\alpha'(s_i))(x_i-x_{i-1})$

And continuity of $\alpha'$ tells us that as we pick the partitions finer and finer, we’ll squeeze $s_i$ and $t_i$ together, and so $\alpha'(t_i)-\alpha'(s_i)$ goes to zero. So, when the integrator $\alpha$ is continuously differentiable, the Riemann-Stieltjes integral reduces to a Riemann integral

$\displaystyle\int\limits_{[a,b]}f(x)d\alpha(x)=\int\limits_{[a,b]}f(x)\alpha'(x)dx$

Now let’s go back to the fence story from yesterday. There’s really some function $g(p)$ that tells us the height of the fence at position $p$ . We got our integrator from the function $p=\alpha(t)$ , which takes a time $t$ and gives the position $p$ at that time. Thus our function giving height at time $t$ is the composition $f(t)=g(\alpha(t))$ . We also note that as time goes from $a$ to $b$ , we walk from $\alpha(a)$ to $\alpha(b)$ . If we put all this into the above equation we find

$\displaystyle\int\limits_{[\alpha(a),\alpha(b)]}g(p)dp=\int\limits_{[a,b]}g(\alpha(t))\alpha'(t)dt$

which is just our change of variables formula.

So here’s what this formula means: we have a real-valued function on some domain — here it’s the height of the fence as a function of position along the ground. We take a region in the space of these variables — the section of the fence we’re walking past — and we “parametrize” it by describing it with a (sufficiently smooth) function taking a single real variable — the position function $p=\alpha(t)$ . We “pull back” our function by composing it with this parametrization, giving us a real-valued function of a real variable, which we can integrate.

The deep thing here is that we have two different parametrizations. We can describe position as $p$ meters from a fixed starting point, or we can describe it as the point we’re passing at $t$ seconds from a certain starting time. In fact, as we change our function $\alpha$ we get all sorts of different parametrizations of the same stretch of ground. And we should measure the same area for the same fence no matter which parametrization we choose.

Given a parametrization $\alpha$ , the integral in our recipe will be the Riemann-Stieltjes integral

$\displaystyle\int\limits_{[a,b]}g(\alpha(t))d\alpha(t)$

which we can reduce to the Riemann integral

$\displaystyle\int\limits_{[a,b]}g(\alpha(t))\alpha'(t)dt$

and it will be the same answer as if we used the “natural” parametrization

$\displaystyle\int\limits_{[\alpha(a),\alpha(b)]}g(p)dp$

And that’s why the change of variables works.

February 29, 2008 - Posted by John Armstrong | Analysis, Calculus

15 Comments »

Do you have any secret plans or motivations for introducing the Riemann-Stieltjes integral, e.g., as key of entry into other more advanced topics in real and functional analysis?

Comment by Todd Trimble | February 29, 2008 | Reply
I don’t have any particular plans. I just find it’s an important stepping stone towards real understanding of integration, and one that’s too often skipped over in the interests of time. In particular, I think that having it down helps understand what’s going on when we move to a measure-theoretic viewpoint.

Comment by John Armstrong | February 29, 2008 | Reply
It’s kinda spooky, but since your post on Integration by Parts your posts are lining up almost spot on with the lectures in my Advanced Calc class. I had to miss today’s lecture (long story…) but I’m almost sure that we’re leading into Riemann-Stieltjes integrals and then measure theory. So thanks for the companion postings, they’ve been great. 😀

Comment by Geoff | March 1, 2008 | Reply
Well, if your class is doing Riemann-Stieltjes you’ve got a great class there. Most advanced calculus classes leave it out.

Comment by John Armstrong | March 1, 2008 | Reply
[…] Riemann-Stieltjes Integral III Last Friday we explained the change of variables formula for Riemann integrals by using Riemann-Stieltjes integrals. Today […]

Pingback by The Riemann-Stieltjes Integral III « The Unapologetic Mathematician | March 3, 2008 | Reply
[…] Let’s do one more easy application of the Riemann-Stieltjes integral. We know from last Friday that when our integrator is continuously differentiable, we can reduce to a Riemann […]

Pingback by The Riemann-Stieltjes Integral IV « The Unapologetic Mathematician | March 6, 2008 | Reply
thanks for your write up, really help me grasp the concept. There seems to a lot of parralles to the line integral. am i correct in thinking riemann-stieltjes integral is like a line integral? is there a difference ?

Comment by ipk | February 16, 2010 | Reply
Line integrals, when I get to them, will ultimately be related back to Riemann integrals. Essentially, the Riemann integral is the line integral along a one-dimensional curve in a one-dimensional space.

There will be a generalization of things like the change-of-variables formula which ultimately lets us translate integrals over curves in other spaces to integrals in one-dimensional spaces, which are Riemann integrals.

Comment by John Armstrong | February 16, 2010 | Reply
thanks i’m looking forward to it.

Comment by isaiahperumalla | February 16, 2010 | Reply
Riemann Stieljes integtal is a very vast in terms of applications. But I really want to know of it’s applications.Also consider Sturm Liouville’s problems in DE.

Comment by Mhammad Rahmatullah Ibrahim | May 30, 2010 | Reply
Riemann Stieljes integtal is a very vast in terms of applications. But I really want to know of it’s applications.Also consider Sturm Liouville’s problems in DE.

Comment by Muhammad Rahmatullah Ibrahim | May 30, 2010 | Reply
In the portion in the above post where you show the R-S integral reduces to the R integral provided the integrator is continuously differentiable, would the following proof work?

$\displaystyle \int_a^b f(t)dv(t)=\lim_{|P|\to 0}\sum_{i=1}^n f(\tau_i)[v(t_i)-v(t_{i-1})]$

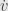Since $\dot{v}$ attains its max on [a,b], 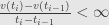$\frac{v(t_i)-v(t_{i-1})}{t_i-t_{i-1}}<\infty$ for all partitions $P$ . This implies

$\displaystyle\begin{aligned}\int_a^b f(t)dv(t)&=\lim_{|P|\to 0}\sum_{i=1}^n f(\tau_i)[v(t_i)-v(t_{i-1})]\\&=\lim_{|P|\to 0}\sum_{i=1}^n f(\tau_i)\frac{v(t_i)-v(t_{i-1})}{t_i-t_{i-1}}(t_i-t_{i-1})\\&=\int_a^b f(t) \frac{dv(t)}{dt}dt\end{aligned}$

as desired.

It's shorter and I've been arguing with someone that tells me step 4 to step 5 is incorrect but can't tell me precisely why this is the case. It makes sense to me because the limit of products is the same as the product of limits. Anyway, if you would weigh-in, I would appreciate it very much.

Comment by Jose | June 2, 2010 | Reply
Okay, Jose, I’ve tried to format your comment a bit, so tell me if I broke anything.

What I’d say is the problem here is you’re trying to take the term

$\frac{v(t_i)-v(t_{i-1})}{t_i-t_{i-1}}$ and have it become the function $\frac{dv(t)}{dt}$ in the limit. The problem is that the term needs to be the evaluation of the function at the sample points of some labelled partition.

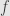In fact, you can be pretty sure that in general you don’t have $\frac{v(t_i)-v(t_{i-1})}{t_i-t_{i-1}}=\frac{dv(\tau_i)}{dt}$ for the same $\tau_i$ where you’ve evaluated $f$ . So what you need to show is that there are always sample points $\xi_i\in[t_{i-1}-t_i]$ so that

$\displaystyle f(\tau_i)\frac{v(t_i)-v(t_{i-1})}{t_i-t_{i-1}}=f(\xi_i)\frac{dv(\xi_i)}{dt}$

for each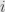 $i$ .

Comment by John Armstrong | June 2, 2010 | Reply
Thank you for your reply. The samples should be the same. I had to think about it. I believe my fallacy was in thinking the limit and the summation could be interchanged as from line 2 to line 3:
\begin{align}
\int_a^b f(x)d\alpha(x)&=\lim_{|x|\to 0}\sum_{i=1}^n f(t_i)[\alpha(x_i)-\alpha(x_{i-1})]\\
&=\lim_{|x|\to 0}\sum_{i=1}^n f(t_i)\frac{\alpha(x_i)-\alpha(x_{i-1})}{x_i-x_{i-1}}(x_i-x_{i-1})\\
&=\sum_{i=1}^n \lim_{|x|\to 0} f(t_i)\frac{\alpha(x_i)-\alpha(x_{i-1})}{x_i-x_{i-1}}(x_i-x_{i-1})\\
&=\sum_{i=1}^n \lim_{|x|\to 0} f(t_i)(x_i-x_{i-1})\lim_{|x|\to 0}\frac{\alpha(x_i)-\alpha(x_{i-1})}{x_i-x_{i-1}}\\
&=\lim_{|x|\to 0}\sum_{i=1}^n f(t_i)(x_i-x_{i-1})\lim_{|x|\to 0}\frac{\alpha(x_i)-\alpha(x_{i-1})}{x_i-x_{i-1}}\\
&=\int_a^b f(x) dx\, \alpha ‘(x)
\end{align}

Thanks again!

Comment by Jose | June 3, 2010 | Reply
- Yes, exactly. To get the sample points, you need to pull part of the limit inside the sum. You can’t just take the limit of the difference quotient to get the derivative without taking the whole limit at once.
  
  Comment by John Armstrong | June 3, 2010 | Reply

About this weblog

This is mainly an expository blath, with occasional high-level excursions, humorous observations, rants, and musings. The main-line exposition should be accessible to the “Generally Interested Lay Audience”, as long as you trace the links back towards the basics. Check the sidebar for specific topics (under “Categories”).

I’m in the process of tweaking some aspects of the site to make it easier to refer back to older topics, so try to make the best of it for now.

RSS Feeds

RSS - Posts
RSS - Comments
Feedback

Got something to say? Anonymous questions, comments, and suggestions at Formspring.me!
Subjects

Subjects
Archives

February 2008

M T W T F S S

1 2 3

4 5 6 7 8 9 10

11 12 13 14 15 16 17

18 19 20 21 22 23 24

25 26 27 28 29

« Jan Mar »
Search for:

The Unapologetic Mathematician

Mathematics for the interested outsider