Indefinite Integrals
We can use integrals to make a set function out of any integrable function
.
We call this set function the “indefinite integral” of , and it is defined for all measurable subsets
. This isn’t quite the same indefinite integral that we’ve worked with before. In that case we only considered functions
, picked a base-point
, and defined a new function
on the domain. In our new language, we’d write
, so the two concepts are related but they’re not quite the same.
Anyhow, the indefinite integral is “absolutely continuous”. That is, for every
there is a
so that
for all measurable
with
. Indeed, if
is an upper bound for
, then we can show that
And so if we make small enough we can keep
small.
Further, an indefinite integral is countably additive. Indeed, if
is a characteristic function then countable additivity of
follows immediately from countable additivity of
. And countable additivity for general simple functions
is straightforward by writing each such function as a finite linear combination of characteristic functions.
With the exception of this last step, nothing we’ve said today depends on the function being simple, and so once we generalize our basic linearity and order properties we will immediately have absolutely continuous indefinite integrals.

I been following your series of posts on measure and integrals. This is getting really interesting now, thanks for taking the time to put these posts up.
I’m not sure why this called an indefinite integral though, considering E is a subset of X
Glad to do it, ip, and glad you’re enjoying them.
As for “indefinite integral”, the idea is just as you said yesterday: “integrating” a function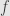 gives us a set function. Once you specify which set to integrate over, you get a number. In the same way, when we consider Riemann integrals we have to specify what interval to integrate over to actually get a number.
gives us a set function. Once you specify which set to integrate over, you get a number. In the same way, when we consider Riemann integrals we have to specify what interval to integrate over to actually get a number.
The old “indefinite integral” is a special, restricted form of the new version, where the only variation in the region is the choice of one endpoint.
[…] Integrals and Convergence I Let’s see how the notion of an indefinite integral plays with sequences of simple functions in the […]
Pingback by Indefinite Integrals and Convergence I « The Unapologetic Mathematician | May 31, 2010 |
I think that you want to write “measurable subsets E \subset X” in the second sentence. Great series of posts.
Thanks emile. Fixed.
[…] their indefinite integrals converge to the same limiting set function. That is, if and are the indefinite integrals of and […]
Pingback by Indefinite Integrals and Convergence II « The Unapologetic Mathematician | June 1, 2010 |
[…] is the indefinite integral of , and is the indefinite integral of . Then if we use to define the integral of we […]
Pingback by Integrable Functions « The Unapologetic Mathematician | June 2, 2010 |
[…] if an integrable function is a.e. nonnegative, then its indefinite integral is […]
Pingback by Properties of Integrable Functions « The Unapologetic Mathematician | June 3, 2010 |
[…] measure, and we’ve shown that convergence in mean implies uniform absolute continuity of the indefinite integrals. All we need to show in the first direction is that if converges in mean to , then the indefinite […]
Pingback by Equicontinuity, Convergence in Measure, and Convergence in Mean « The Unapologetic Mathematician | June 9, 2010 |
[…] this is a statement about finite measure spaces; the function is here so that the indefinite integral of will give us a finite measure on the measurable space to replace the (possibly non-finite) […]
Pingback by The Integral Mean Value Theorem « The Unapologetic Mathematician | June 14, 2010 |
[…] the Integral Given an integrable function , we’ve defined the indefinite integral to be the set […]
Pingback by Extending the Integral « The Unapologetic Mathematician | June 21, 2010 |
[…] The Jordan Decomposition of an Indefinite Integral So, after all our setup it shouldn’t be surprising that we take an integrable function and define its indefinite integral: […]
Pingback by The Jordan Decomposition of an Indefinite Integral « The Unapologetic Mathematician | June 29, 2010 |
[…] Continuity I We’ve shown that indefinite integrals are absolutely continuous, but today we’re going to revise and extend this notion. But first, to review: we’ve […]
Pingback by Absolute Continuity I « The Unapologetic Mathematician | July 1, 2010 |
[…] Continuity II Now that we’ve redefined absolute continuity, we should tie it back to the original one. That definition makes precise the idea of “smallness” as being bounded in size below […]
Pingback by Absolute Continuity II « The Unapologetic Mathematician | July 2, 2010 |
[…] let be a -finite signed measure on which is absolutely continuous with respect to . Then is an indefinite integral. That is, there is a finite-valued measurable function so […]
Pingback by The Radon-Nikodym Theorem (Statement) « The Unapologetic Mathematician | July 6, 2010 |
[…] define a signed measure as the indefinite integral of . Immediately we know that is totally -finite and that . And, obviously, is the Radon-Nikodym […]
Pingback by Corollaries of the Chain Rule « The Unapologetic Mathematician | July 13, 2010 |
Nice Site; I’ve learned a few things in just this read!. Re the proof of absolute continuity, what do you do if f is not bounded in E;
f may even take the value oo there, right (albeit in a subset of E of measure zero)? How would the argument c*m(E)->0 ; where c is
an upper-bund proceed then?.
To be honest I’m not sure offhand. I’m not really an analyst by training.