Bounded Variation addenda
I left a few things out of last Saturday’s post. Since I’ve spent all morning finishing off that paper (I’ll post the arXiv link tomorrow when it shows up) I’m sort of mathed out for the moment. I’ll just tack up these addenda and take a nap or something to brace for tomorrow’s Clifford Lectures (which is the only day of them I’ll be able to see, due to airline pricing).
Okay, so we said that we can represent any function 
Usually nonuniqueness gets messy, but there’s a way this can come in handy. If we pick 
It also turns out that wherever the function 
Now let’s look at bounded increasing functions for a moment. Such a function 
So increasing functions can only have a countable number of jump discontinuities. But any function of bounded variation is the difference of two increasing functions. Thus any function of bounded variation can only have a countable number of discontinuities, where at worst the function jumps up or down by a finite amount at each one. The only other sort of discontinuity is a point where the function has a limit, but takes a different value. For instance, 
8 Comments »
Leave a reply to eugene lalonde Cancel reply
RSS Feeds
The Unapologetic Mathematician 
John,
Thank you for your very interesting stuff on bdd- variation.
My question is : Say f and g are 2 monotonically increasing
functions. Is f-g mono increasing or decreasing. In my
case it looks as if f-g is decreasing. Is there any
proof of what f-g has to be ?
How do you determine the number that is the total variation
of f-g ?
Eugene
The best you can say for that difference is that it’s of bounded variation. It might be increasing in some areas and decreasing in others. As for the variation of the difference, the best you can say is that it’s less than the sum of the variations of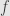
and 
separately. Luckily, those variations are easy, since for monotonic functions it’s just the difference in values at the two endpoints.
[…] consider jump discontinuities. These are especially useful to understand, since they’re the only sort a function of bounded variation can have. So let’s say we take an interior point and define as simple a function as we can with a […]
Pingback by Integrating Across a Jump « The Unapologetic Mathematician | March 14, 2009 |
Hi! Thanks for posting such interesting stuff. I would like to be able to say that all discontinuity points of a bounded variable function are isolated. Your observation “since each jump must increase by a finite amount, we can only have a countable number of them in a finite interval, or else the function would have to take arbitrarily large values and would no longer be bounded” would be sufficient. But… are you sure it is true? (I am not sure). Any reference? Thanks!
… Sorry, my previous comment is nonsense. My real problem is: I would like to order the set of discontinuities of a bounded-variation function , i.e. to put them in a monotone sequence. Do you think that can be done?
I’m not sure what you mean. They’re already ordered because they lie on the real line, which is totally ordered.
Sorry, I didn’t explain well. Given a bounded variation function $f$, I would like to say “let $(x_i)$ be the sequence of discontinuity points of $f$, with $x_i < x_{i+1}$ for all $n \in \mathbb N$." But I don't see if that can be done. For example, the set of discontinuity points can be $\{1/n | n \in \mathbb N}$. Or worse, $\{1/n+1/m | m,n \in \mathbb N}$ (which is also countable), in which every point is an accumulation point. Can you order those points in an increasing sequence? I do not see how there is a first (i.e. lowest) element, or a second, or… So it seems it can´t be done in general. What do you think?
You’re asking, in effect, if the order induced by the order on the real line (the one I was talking about) is a well-order. And your examples show that, no, in general it’s not.