The Fundamental Theorem of Line Integrals
At last we can state one of our special cases of Stokes’ theorem. We don’t need to prove it, since we already did in far more generality, but it should help put our feet on some familiar ground.
So, we take an oriented curve in a manifold
. The image of
is an oriented submanifold of
, where the “orientation” means picking one of the two possible tangent directions at each point along the image of the curve. As a high-level view, we can characterize the orientation as the direction that we traverse the curve, from one “starting” endpoint to the other “ending” endpoint.
Given any -form
on the image of
— in particular, given an
defined on
— we can define the line integral of
over
. We already have a way of evaluating line integrals: pull the
-form back to the parameter interval of
and integrate there as usual. But now we want to use Stokes’ theorem to come up with another way. Let’s write down what it will look like:
where is some
-form. That is: a function. This tells us that we can only make this work for “exact”
-forms
, which can be written in the form
for some function
.
But if this is the case, then life is beautiful. The (oriented) boundary of is easy: it consists of two
-faces corresponding to the two endpoints. The starting point gets a negative orientation while the ending point gets a positive orientation. And so we write
That is, we just evaluate at the two endpoints and subtract the value at the start from the value at the end!
What does this look like when we have a metric and we can rewrite the -form
as a vector field
? In this case,
is exact if and only if
is “conservative”, which just means that
for some function
. Then we can write
which should look very familiar from multivariable calculus.
We call this the fundamental theorem of line integrals by analogy with the fundamental theorem of calculus. Indeed, if we set this up in the manifold , we get back exactly the second part of the fundamental theorem of calculus back again.

[…] Stokes’ theorem that people learn with that name in calculus courses. Ironically, unlike the fundamental theorem and divergence theorem special cases, Stokes’ theorem only works in dimension , where the […]
Pingback by The Classical Stokes Theorem « The Unapologetic Mathematician | November 23, 2011 |
[…] vector fields. Indeed, if we have a curve that starts at a point and ends at a point then fundamental theorem of line integrals makes it easy to […]
Pingback by Conservative Vector Fields « The Unapologetic Mathematician | December 15, 2011 |
Excellent site; nice job!.
I hope this is not too simple-minded. The last part of this segment seems to say , with the expression f(c(1))-f(c(0)) , that the line
integral of f along c, is independent of the path joining c(1) with c(0) in the manifold. But I believe this independence of path is true
only when the manifold M is simply-connected, right? Equivalently, the integral depends only on the homotopy class ( with fixed
endpoints c(1),c(0)) of the curve joining the endpoints, so that, if M is simply-connected, any two curves are homotopic, but this is
not so if M is not simply-connected. Could you please tell me where the assumption of simple-connectedness is made in your post–
if it is made at all?
Thanks, and keep up with the nice site.
@genios:
The extra assumption that makes path-independence work is not (in this case) about the space (i.e. simple-connectedness), but about the thing being integrated – it must be the gradient of something.
(I hope I’m not wrong.)
@Andrei: I think you’re right. My comment comes from something I remember about homology, re closed curves defined in a region R of the complex plane, where C~C’ (homologous) , if , given f analytic in R, we had Int_C fdx =Int_C’fdx if there is only one class, then R is homologically-trivial. I think if R is not simply-connected, then there is at least one form w defined in R that is not exact. So maybe I should
change my statement to saying that if R is simply-connected, then not all forms are exact.
Yes, exactly. It’s more about the homology than the homotopy type of the region, but nontrivial first homotopy does imply nontrivial first homology.
So, where does the assumption of trivial homology come in? It’s actually sort of deep, and comes from the relationship between first homology defined in terms of curves in the region and first cohomology defined in terms of 1-forms. The key assumption is that in our region we can write our 1-form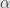 as
as 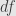 for some function
for some function 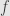 .
.
To see how this matters, think about the real plane with the origin removed. We can define a vector field wrapping around the origin like
which, locally, is the gradient of the “angle function”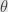 . We can integrate this around the unit circle and get a nonzero answer when we get back to the place where we started, seemingly in violation of the fundamental theorem of line integrals.
. We can integrate this around the unit circle and get a nonzero answer when we get back to the place where we started, seemingly in violation of the fundamental theorem of line integrals.
But the problem is that we can’t define the angle function consistently on our whole region! There is no single differentiable function on the region whose gradient is our given vector field. And the ability to define such a vector field is deeply connected to the fact that it has a hole around which we can wrap a closed curve.