Functions of Bounded Variation I
In our coverage of the Riemann-Stieltjes integral, we have to talk about Riemann-Stieltjes sums, which are of the form
How can we choose a function to make sums like this get really big? Well, we could make the function
get big, but that’s sort of a cheap trick. Let’s put a cap that
. Now how can we make a Riemann-Stieltjes sum big?
Well, sometimes and sometimes
. In the first case, we can try to make
, and in the second we can try to make
. In either case, we’re adding as much as we possibly can, subject to our restriction.
We’re saying a lot of words here, but what it boils down to is this: given a partition we can get up to in our sum. As we choose finer and finer partitions, it’s not hard to see that this number can only go up. So, if there’s an upper bound
for our
, then we’re never going to get a Riemann-Stieltjes sum bigger than
. That will certainly make it easier to get nets of sums to converge.
So let’s make this definition: a function is said to be of “bounded variation” on the interval
if there is some
so that for any partition
we have
That is, is an upper bound for the set of variations as we look at different partitions of
. Then, by the Dedekind completeness of the real numbers, we will have a least upper bound
, which we call the “total variation” of
on
Let’s make sure that we’ve got some interesting examples of these things. If is monotonic increasing — if
implies that
— then we can just drop the absolute values here. The sum collapses, and we’re just left with
for every partition. Similarly, if
is monotonic decreasing then we always get
. Either way,
is clearly of bounded variation.
An easy consequence of this condition is that functions of bounded variation are bounded! In fact, if has variation
on
, then
for all
. Indeed, we can just use the partition
and find that
from which the bound on easily follows.

The strict inequality |f|<1 later turns out to be not so strict in your post.
Thanks for catching that.
[…] of Bounded Variation II Let’s consider the collection of functions of bounded variation on a little more deeply. It turns out that they form a subring of the ring of all real-valued […]
Pingback by Functions of Bounded Variation II « The Unapologetic Mathematician | March 6, 2008 |
[…] continue our study of functions of bounded variation by showing that total variation is “additive” over its interval. That is, if is of […]
Pingback by Functions of Bounded Variation III « The Unapologetic Mathematician | March 9, 2008 |
[…] over subintervals As I noted when I first motivated bounded variation, we’re often trying to hold down Riemann-Stieltjes sums to help them converge. In a sense, […]
Pingback by Integrability over subintervals « The Unapologetic Mathematician | March 21, 2008 |
[…] Oscillation in a function is sort of a local and non-directional version of variation. If is a bounded function on some region , and if is a nonempty subset of , then we define the […]
Pingback by Oscillation « The Unapologetic Mathematician | December 7, 2009 |
[…] explicitly, let be a continuous function on the rectangle , and let be of bounded variation on . Define the function on […]
Pingback by Continuity of Partial Integrals « The Unapologetic Mathematician | January 12, 2010 |
Why is the upper bound M on alpha, and not on the set of all possible “distances” (i.e. absolute values of differences) between successive alpha values?
It may not be completely clear; if there’s an upper bound on the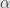 values then there’s an upper bound on their differences. That’s in the motivation, but the actual definition that follows is written in terms of an upper bound on the sums of absolute differences.
values then there’s an upper bound on their differences. That’s in the motivation, but the actual definition that follows is written in terms of an upper bound on the sums of absolute differences.
Thank you for the prompt response and clarification. In the motivation, do the alpha values need to be bounded below as well (or is there some property of the alpha values that I am missing, like non-negativity)? I do apologise if I am missing something obvious. Thanks.
Yeah, they’d probably need to be bounded above and below; the motivation is meant to give the idea, but the definition is precise.
Thank you